I posted awhile ago about how I used paper folding to help my students discover the Triangle Midsegment Theorem. Then, I had my students glue their folded triangles into their interactive notebooks. I had them fold altitudes, and angle bisectors as well.
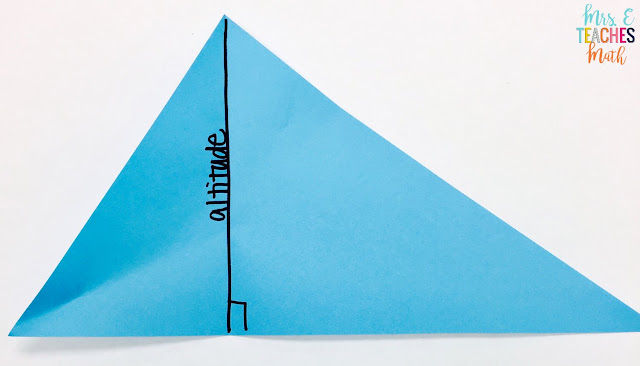
Altitudes
I pre-cut obtuse triangles, right triangles, and acute triangles. It didn’t take me very long. I pretty much just hacked at a stack of paper. I passed them out and told students to fold the triangle so that a side overlaps itself and the fold contains the opposite vertex. At this point, I had only given students the definition of an altitude.
Then, I had them open up their fold and draw the line that they had drawn. I asked them if they noticed anything interesting about the line. Someone noticed that it was perpendicular, and so we included the right angle on the triangle. I also had them try to fold the altitude for the other sides. Some of them had trouble, and we talked about how the altitude can be outside or inside the triangle.
Angle Bisectors
Again, I pre-cut obtuse, right, and acute triangles. I gave each student a triangle (they were all different). I told them to fold the triangle so that the opposite sides meet and contain the vertex.
I had them do this for each of the angles in the triangle. Once they were finished, I had them trace all of the folds they had made. Then, they marked the point of concurrency.
Paper folding this way was an interesting way to think about constructions, without actually doing constructions and using protractors and compasses, etc. I will do this again next year. I thought about having pre-made samples to show students to make the activity go faster, but I think some of the discovery would then be lost.